Snell’s law plays a crucial role in the study of optics, particularly in understanding how light behaves when it travels through different media. It is also known as the law of refraction.
This law explains the relationship between the angles of incidence and refraction, allowing scientists and engineers to predict how light will bend as it moves from one material, like air, to another, such as water or glass.
The discovery of Snell’s law dates back to 1621, attributed to the Dutch astronomer Willebrord Snell. By delving into this concept, readers encounter a fundamental principle that underpins many optical applications, from glasses and cameras to fiber optics.
Understanding the various names associated with this law enriches one’s perspective on how light interacts with the world around us.
As readers explore Snell’s law, they will uncover its significance not only in theory but also in practical applications. The exploration of this scientific principle opens doors to a deeper appreciation of light’s journey through different media and its impact on everyday life.
Fundamentals of Snell’s Law
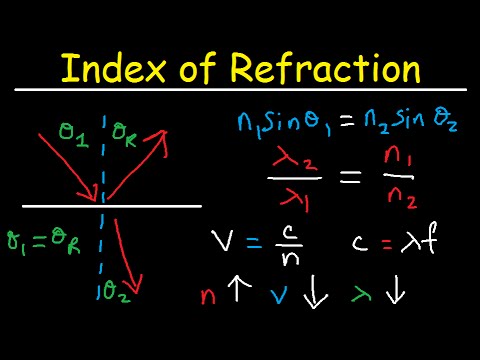
Snell’s Law describes how light rays change direction when they pass through different materials. It involves key concepts such as the angle of incidence, angle of refraction, and refractive index, which are essential for understanding how light behaves in various media.
Understanding Refraction
Refraction is the bending of light as it travels from one medium to another. When a light ray hits the boundary between two materials, it changes speed, causing a shift in direction.
This interaction is important in many optical devices, including lenses and glasses.
The angle at which the light hits the surface is called the angle of incidence. The angle at which it bends is known as the angle of refraction. The normal line, which is perpendicular to the boundary, helps measure these angles.
The ratio of these angles is a fundamental aspect of Snell’s Law.
The Role of the Refractive Index
The refractive index is a measure of how much a material can bend light. Each material has its own refractive index, which affects the light’s speed. For example, light travels slower in water than in air.
Snell’s Law reveals that the ratio of the sine of the angle of incidence to the sine of the angle of refraction equals the ratio of the refractive indices of the two media.
This relationship can be expressed mathematically as:
[
n_1 \sin(\theta_1) = n_2 \sin(\theta_2)
]
Where ( n_1 ) and ( n_2 ) are the refractive indices, and ( \theta_1 ) and ( \theta_2 ) are the angles of incidence and refraction, respectively. Understanding this principle is crucial for predicting how light behaves when transitioning between different substances.
Mathematical Expression and Derivation

Snell’s Law provides a crucial mathematical framework for understanding how light behaves when it passes through different mediums. This section presents the equation associated with Snell’s Law and explores how it is derived.
Snell’s Law Equation
The equation for Snell’s Law is expressed as:
[ n_1 \sin(\theta_1) = n_2 \sin(\theta_2) ]
In this equation:
- ( n_1 ) and ( n_2 ) represent the refractive indices of the two different media.
- ( \theta_1 ) is the angle of incidence, the angle between the incoming light ray and the normal line at the boundary.
- ( \theta_2 ) is the angle of refraction, the angle between the refracted ray and the normal.
Willebrord Snell discovered this relationship in 1621, highlighting how light bends at the interface between materials with different densities. As light moves from one medium to another, its speed changes due to differing refractive indices, which leads to a change in direction.
Deriving Snell’s Law
To derive Snell’s Law, consider the principles of wavefronts and the speed of light in different media. When light strikes a boundary, the change in speed causes it to refract.
Assuming light travels faster in air than in water, the speed of light in each medium can be defined as:
- ( v_1 ) in the first medium (e.g., air).
- ( v_2 ) in the second medium (e.g., water).
Using the relationship ( n = \frac{c}{v} ) (where ( c ) is the speed of light in vacuum), the refractive indices are defined as:
- ( n_1 = \frac{c}{v_1} )
- ( n_2 = \frac{c}{v_2} )
By applying the sine rule in the context of the triangle formed by the incident and refracted rays, it leads to the conclusion ( n_1 \sin(\theta_1) = n_2 \sin(\theta_2) ).
Thus, the mathematical expression of Snell’s Law demonstrates the predictable behavior of light as it travels through different materials.
Applications and Implications of Snell’s Law

Snell’s Law plays a crucial role in various applications related to optics. Its principles are essential for understanding how light interacts with different media. This section will explore its importance in optical instruments and the field of fiber optics.
Optical Instruments and Lenses
Snell’s Law is fundamental in the design of optical instruments, such as microscopes, binoculars, and telescopes. It explains how light bends when it passes through different lenses. Each lens has a specific refractive index that determines how much light is refracted.
For example, in a microscope, light entering the objective lens changes direction based on the angle of incidence and the lens material. This bending of light allows for magnified views of small objects.
Proper understanding of Snell’s Law helps in creating lenses with the desired focal length and clarity. Devices like microscopes rely on these principles for effective imaging.
Total Internal Reflection and Fiber Optics
Another significant application of Snell’s Law is in fiber optics, where it describes total internal reflection. When light travels through an optical fiber, it must hit the boundary at an angle greater than the critical angle to reflect completely within the fiber.
This principle enables efficient data transmission over long distances. The refractive index difference between the fibers and the surrounding medium plays a critical role in maintaining signal strength.
In fiber optics, isotropic media surround the fibers, optimizing their performance. Innovations in this field continue to change communication technology, making it faster and more reliable.
Historical Context and Future Directions

The exploration of Snell’s law has shaped the field of optics both historically and in contemporary studies. Key figures like Willebrord Snellius laid the groundwork for understanding light behavior, while modern advancements continue to expand its applications and significance.
Contributions of Willebrord Snellius
Willebrord Snellius, a Dutch mathematician and astronomer, is credited with formulating the law of refraction around 1621. His work established a mathematical relationship between the angle of incidence and the angle of refraction as light passes through different media.
Snellius’s findings were pivotal in the study of optics. Prior to his discoveries, light behavior was not well understood. Building on earlier work by Greek and Arab scholars, he provided a clearer framework that influenced later scientists.
The law, named after him, has become essential in numerous applications, from lens design to navigation. His contributions have paved the way for further research, inspiring scientists like Christiaan Huygens, who developed concepts that expanded on Snellius’s initial findings.
Advancements in Optics
The legacy of Snellius extends into modern optics.
Breakthroughs in this field continue to enhance technology. Innovations like fiber optics and advanced imaging systems are direct results of the principles established by Snell’s law.
Contemporary researchers are exploring new materials and technologies to manipulate light even further.
The development of metamaterials, for instance, allows for the creation of lenses that can bend light in unprecedented ways.
As the field progresses, future advancements promise to improve communication, medical imaging, and various optical devices.
The ongoing exploration of Snell’s principles continues to reveal new potentials in understanding and utilizing light, maintaining the relevance and adaptability of Snell’s contributions in the scientific community.

