In the study of optics, understanding how to draw the angle of incidence is essential.
To draw the angle of incidence, one must create a clear representation that shows the incoming light ray, the surface it strikes, and the angle formed with a normal line perpendicular to that surface.
This foundational concept leads to deeper insights into reflection and refraction, which are vital in various applications, from designing optical devices to understanding everyday phenomena.
The angle of incidence is not just a theoretical idea; it plays a crucial role in the law of reflection, which states that the angle of incidence equals the angle of reflection. When light rays hit a surface, they behave predictably, and this predictability is governed by Snell’s law when refraction occurs.
Understanding these principles allows one to visualize and calculate how light interacts with different materials.
Drawing the angle of incidence is straightforward yet involves key elements that connect with broader optical concepts. By mastering this skill, one opens doors to exploring more complex optical behaviors and applications, making it a valuable addition to anyone’s knowledge in the field.
Understanding Light and Angles

Light behaves in a specific manner that is essential in understanding concepts such as the angle of incidence. This section discusses the nature of light rays, defines the angle of incidence, and highlights the significance of the normal line in optics.
Nature of Light Rays
Light rays are straight lines that represent the path of light as it travels. In optics, these rays are essential for understanding how light interacts with surfaces.
When a light ray strikes a surface, it can be reflected or refracted.
Each light ray travels in a direction determined by its angle relative to various surfaces. The behavior of these rays is described by fundamental laws like the law of reflection, which states that the angle of incidence equals the angle of reflection. This principle helps in practical applications, such as designing optical instruments. Learning more about light can be pursued through various articles.
Defining the Angle of Incidence
The angle of incidence is formed between an incoming light ray and the normal line. The normal line is an imaginary line that is perpendicular to the surface at the point of contact. This angle is crucial in optics as it affects how light behaves when it encounters different media.
In equations, the angle of incidence is often denoted as “θi.” When light hits a reflective surface, this angle plays a key role in determining the angle at which it reflects. Understanding this relationship is important for various applications involving light, from designing mirrors to analyzing lenses.
Normal Line and Its Significance
The normal line serves as a reference point for measuring angles in optics. It is drawn perpendicular to the surface where the light ray strikes.
The significance of the normal line lies in its consistency across different surfaces, providing a clear means to define angles of incidence and reflection.
Using the normal line allows for precise calculations in optical designs. By accurately defining θi with respect to the normal, engineers and scientists can predict how light will reflect or refract at boundaries. This understanding is fundamental in creating effective optical devices, enhancing applications in technology and research.
Laws Governing Light Interaction
Light behavior is governed by specific laws that explain how it interacts with different surfaces and mediums. These laws help to understand both reflection and refraction, essential concepts in optics.
Exploring the Law of Reflection
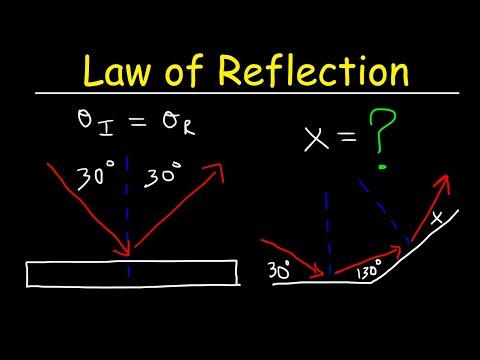
The law of reflection states that the angle of incidence is equal to the angle of reflection. This means that when light hits a reflective surface, the angle at which it arrives is the same as the angle at which it leaves. The incident ray, the reflected ray, and the normal (a line perpendicular to the surface) all lie in the same plane.
In practical terms, if a light ray strikes a mirror at 30 degrees to the normal, it will reflect off at 30 degrees as well. This relationship is fundamental in designing reflective devices such as mirrors and periscopes. Understanding this law is crucial for accurately drawing and measuring angles of incidence and reflection in various applications.
Understanding Refraction of Light
Refraction occurs when light passes from one medium to another, changing speed and direction. According to Snell’s Law, the relationship between the angle of incidence and angle of refraction is defined mathematically: n₁ sin(i) = n₂ sin(r). Here, n₁ and n₂ are the refractive indices of the two mediums, while i and r are the angles of incidence and refraction, respectively.
For example, when light travels from air (n ≈ 1) into water (n ≈ 1.33), it slows down and bends towards the normal. This bending effect is why a straw in a glass of water looks broken. Understanding refraction helps in applications like lens design and optical fibers.
Effect of Mediums on Light Behavior
Different mediums affect how light travels and behaves. Each medium has a specific refractive index that impacts speed.
For example, glass has a much higher refractive index than air, resulting in greater bending of light.
When light moves through varying mediums, such as air to water, the deviation of light rays depends on the refractive indices. This principle plays a crucial role in devices like eyeglasses and cameras. Knowing how the medium influences light is essential for creating effective optical instruments and understanding real-world phenomena.
Practical Guide to Drawing Angles of Incidence

Understanding how to draw the angle of incidence accurately is essential in optics. This guide provides clear steps to ensure precise measurements, outlines the process of drawing incident angles, and describes how to identify reflected and refracted rays.
Using a Protractor for Accurate Measurements
A protractor is a vital tool for measuring angles, including the angle of incidence. It typically has a semicircular shape marked from 0 to 180 degrees.
To measure an angle:
- Place the Center: Align the protractor’s center hole with the point where the ray hits the surface.
- Align the Base Line: Ensure the baseline of the protractor is along the incident ray.
- Read the Angle: Where the second ray meets the protractor’s scale gives the angle of incidence.
Using a protractor correctly ensures accurate and consistent angle readings, which is crucial for further calculations in optics, such as determining reflection and refraction.
Step-by-Step Process for Drawing Incident Angles
To draw the angle of incidence, follow these systematic steps:
- Draw the Surface: Start by sketching the boundary surface where light will hit.
- Mark the Normal Line: Draw a dashed line perpendicular to the surface. This represents the normal line.
- Choose Your Angle: Decide on the angle of incidence. Common angles are 30°, 45°, or 60°.
- Use the Protractor: Position the protractor to measure this angle accurately from the normal line.
- Draw the Incident Ray: From the surface, draw the incident ray at the chosen angle toward the normal.
These steps create a visual representation of how light interacts with different surfaces, critical for understanding reflection and refraction.
Identifying Reflected and Refracted Rays
Once the incident ray is drawn, identifying the reflected and refracted rays is the next step.
Reflected Ray:
- The reflected ray bounces off the surface.
- According to the law of reflection, the angle of reflection equals the angle of incidence.
Refracted Ray:
- The refracted ray passes into the new medium, bending at the interface.
- The angle of refraction can be calculated using Snell’s Law, which relates the angles to the index of refraction of the two media.
Clearly marking these rays provides vital information for studying light behavior, allowing the exploration of concepts like Snell’s Law and total internal reflection.
Complex Concepts in Light Incidence
Light incidence involves various concepts that deepen the understanding of how light interacts with different surfaces. Key ideas include total internal reflection, the critical angle, and how the speed and color of light influence these interactions.
Total Internal Reflection and Critical Angle
Total internal reflection occurs when light travels from a denser medium to a less dense medium and hits the boundary at a specific angle, known as the critical angle. If the angle of incidence exceeds this critical angle, the light is entirely reflected back into the denser medium.
The critical angle can be calculated using Snell’s Law. For example, if light travels from water (n ≈ 1.33) to air (n ≈ 1.00), the critical angle θc can be found with the formula:
sin(θc) = n2/n1
Where n2 is the refractive index of air, and n1 is that of water. For this case, the critical angle is about 48.6 degrees.
Mathematical Relationships in Refraction
Refraction is the bending of light as it passes from one medium to another. The angles of incidence and refraction are linked by Snell’s Law, which states:
n1 * sin(θ1) = n2 * sin(θ2)
Here, n1 and n2 are the refractive indices of the first and second mediums. The angle θ1 is the angle of incidence, while θ2 is the angle of refraction. This relationship allows for predicting how much light will bend when entering a new medium.
For instance, when light moves from air into glass, the angle will reduce, showing how refractive indices impact light direction and behavior.
Color and Speed of Light Considerations
The speed of light changes when it travels through different materials, affecting its color perception.
Light consists of multiple wavelengths, each corresponding to different colors.
When light enters a denser medium, it slows down, causing the different colors to bend at varying angles due to their wavelengths. This phenomenon leads to dispersion, which explains why prisms separate white light into a spectrum of colors.
Understanding how color and the speed of light interact enhances the comprehension of optical effects in lenses and crystals.
Observing these interactions reveals precise behaviors crucial for fields like photography, optics, and even astronomy.
