Understanding how to find the angle of incidence is crucial in the study of optics, especially when analyzing how light interacts with different surfaces.
The angle of incidence is defined as the angle between the incoming ray of light and a line perpendicular to the surface at the point of contact. This concept is essential for both reflection, where light bounces off a surface, and refraction, where light bends as it passes through different mediums.
To calculate this angle, one must first identify the normal line, which acts as a reference point. By measuring the angle between the incoming ray and this normal line, one can determine the angle of incidence accurately.
This measurement plays a significant role in various applications, from designing optical instruments to understanding natural phenomena such as rainbows.
As light travels and interacts with various materials, the angle of incidence affects how light behaves. Whether it is a shiny mirror reflecting light or water bending a ray entering from the air, knowing how to calculate this angle is vital for anyone working in optics or simply curious about the science of light.
Fundamentals of Light and Surfaces

Light interacts with different surfaces and mediums, playing a crucial role in how it travels and reflects. Understanding these interactions helps in finding the angle of incidence, which is vital in optics.
Understanding Light Rays and Mediums
A light ray is a straight path that light travels along. Light moves through various mediums, such as air, water, or glass. The speed of light changes depending on the medium; it moves fastest in a vacuum and slows down in denser materials.
When a ray of light hits a surface, it is known as the incident ray. The point where the ray meets the surface is where the angle of incidence is measured. This angle is formed between the incident ray and the surface normal, an imaginary line that is perpendicular to the surface.
Properties of Reflective and Transmissive Surfaces
Reflective surfaces bounce light away, like a mirror. When light strikes such a surface, the angle of incidence equals the angle of reflection. This is known as the law of reflection.
In contrast, transmissive surfaces allow light to pass through. This can result in refraction, where light bends as it enters a new medium. Each type of surface affects how light behaves, altering its path and speed.
Principles of Reflection and Refraction
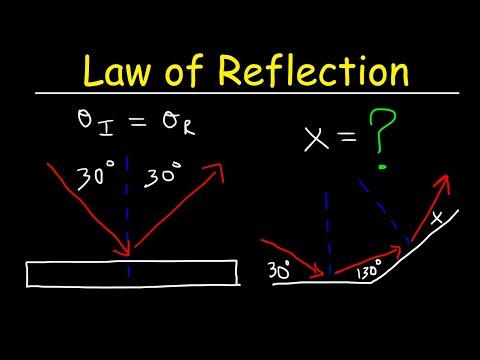
Reflection and refraction are fundamental concepts in optics that explain how light behaves when it interacts with different surfaces. Understanding these principles is essential to grasp the angle of incidence and its effects on light behavior.
Law of Reflection
The law of reflection states that the angle of incidence is equal to the angle of reflection. This means that when a light ray strikes a reflective surface, the angle at which it hits the surface is the same as the angle at which it bounces away.
- Angle of Incidence: The angle between the incoming ray and the normal (an imaginary line perpendicular to the surface).
- Angle of Reflection: The angle between the ray that leaves the surface and the normal.
Both angles are measured from the normal line. This principle can be observed in everyday scenarios, such as when looking into a mirror. The clarity of the reflection depends on the smoothness of the surface involved.
Snell’s Law and Refraction
Refraction occurs when light passes from one medium to another, changing its speed and direction. Snell’s Law can be used to describe this phenomenon mathematically:
n₁ * sin(θ₁) = n₂ * sin(θ₂)
Here:
- n₁ and n₂ are the refractive indices of the two media.
- θ₁ is the angle of incidence, and θ₂ is the angle of refraction.
The refractive index indicates how much light slows down in a medium compared to a vacuum. For example, light travels slower in water than in air, which results in a bending effect when light enters the water.
Critical Angle and Total Internal Reflection
The critical angle is the angle of incidence beyond which light cannot pass through a boundary and is instead reflected back into the medium. It occurs when light travels from a denser medium to a less dense one. The relationship is described by:
sin(θc) = n₂/n₁
If the angle of incidence exceeds the critical angle, total internal reflection occurs. This phenomenon is essential in optical fibers, allowing light to be transmitted over long distances without loss.
Measuring Angles of Incidence

To accurately determine the angle of incidence, it is essential to consider various factors that influence measurement. This includes how angles can vary in different mediums, the significance of refractive indices, and how surface orientation impacts results.
Incidence in Different Mediums
The angle of incidence varies depending on the medium through which light travels. When light passes from air into a denser medium like glass or water, the behavior of the light ray changes.
-
Air to Glass: When light enters glass, it slows down and bends towards the normal line. The angle of incidence is measured from this normal line to the incoming ray.
-
Air to Water: Similar to glass, the angle will decrease as light moves from air to water. This change is essential in calculating the angle of refraction.
Using tools like a protractor or a laser can help measure these angles accurately.
Role of Refractive Indices
Refractive indices are crucial when calculating angles of incidence. Each medium has a specific refractive index, which is the ratio of light’s speed in a vacuum to its speed in that medium.
- Example Values:
- Air: 1.00
- Water: 1.33
- Glass: 1.5
Snell’s Law relates the angles of incidence and refraction through these indices. The formula, n₁ * sin(θ₁) = n₂ * sin(θ₂), allows for easier determination of angles when transitioning between mediums.
Influence of Surface Orientation
The orientation of the surface also impacts the angle of incidence. A surface can be flat, angled, or even curved, changing how light interacts with it.
-
Flat Surfaces: When light strikes a flat surface, the angle of incidence is straightforward to measure. It is simply the angle between the incoming ray and the normal line.
-
Angled Surfaces: For surfaces that are not flat, such as angled or reflective surfaces, the angle needs careful adjustment with respect to the normal. Measuring becomes crucial for applications like reflection and refraction in optics.
Applications in Optics

The angle of incidence is vital in several optical applications, particularly in the design and function of lenses, mirrors, and prisms. Understanding this angle helps improve image quality and light manipulation in various optical devices.
Design of Lenses and Mirrors
Lenses and mirrors rely on the angle of incidence to focus light effectively. When light rays hit a lens, the angle affects how they bend, determining the lens’s focusing power.
For example, in a convex lens, converging light rays will meet at a focal point based on their angle of incidence.
Similarly, mirrors use this angle to reflect light. A plane mirror reflects light at the same angle at which it arrives, known as the angle of reflection. This principle is crucial in creating clear images in devices like microscopes and binoculars, where precise light management ensures optimal performance.
The concept plays a significant role in the overall design of reflective devices, enhancing their utility in fields like photography and astronomy.
Practical Use in Prisms and Reflective Devices
Prisms utilize the angle of incidence to separate light into its color components.
When light enters a prism, the angle at which it strikes the surface dictates how much it bends. This bending creates a spectrum of colors, as seen in rainbows.
Reflective devices, such as spotting scopes, also benefit from understanding this angle.
The design must account for how light reflects off surfaces to avoid distortions in the image. Proper angles enhance visibility and clarity, allowing users to see details more effectively.
The angle’s importance in both prisms and reflective devices exemplifies its fundamental role in practical optics.
